This the code I draw the circle center at `G` and radius `radius = \myr`. I get the circle, but dashed behind the circle incorrect.

```
\documentclass[12pt]{standalone}
\usepackage{tikz,tikz-3dplot}
\usetikzlibrary{fpu,calc}
\usepackage{tikz-3dplot-circleofsphere}
\def\pgfmathsetmacroFPU#1#2{\begingroup%
	\pgfkeys{/pgf/fpu,/pgf/fpu/output format=fixed}%
	\pgfmathsetmacro{#1}{#2}%
	\pgfmathsmuggle#1\endgroup}%
\begin{document}
	\tdplotsetmaincoords{70}{70}
	\begin{tikzpicture}[tdplot_main_coords,line cap=butt,line join=round,c/.style={circle,fill,inner sep=1pt},
		declare function={a=3;b=4;c=5;m=3;n=3;p=3;}]
		\begin{scope}[/pgf/fpu,/pgf/fpu/output format=fixed]
			\path (0,0,0) coordinate (A)
			(c,0,0) coordinate (B)
			({(pow(b,2) + pow(c,2) - pow(a,2))/(2*c)},{sqrt((a+b-c) *(a-b+c) *(-a+b+c)* (a+b+c))/(2*c)},0) coordinate (C)
({c/2},{(c* (a^2 + b^2 - 
	c^2))/(2* sqrt((a + b - c) *(a - b + c)* (-a + b + c)* (a + b + c)))},0) 	coordinate	(G)
			({(c^2 + m^2 - n^2)/(2*c)},{(-c^4 + (a^2 + b^2 + m^2 + n^2 - 2*p^2)*c^2 + (m - n)*(m + n)*(a - b)*(a + b))/(2*sqrt(-(a + b - c)*(a - b + c)*(a - b - c)*(a + b + c))*c)},{-1*sqrt((a - b + c)*(a - b - c)*(a + b + c)*(b^2*n^4 + ((a^2 - b^2 - c^2)*p^2 + (-b^2 + m^2)*c^2 + b^4 + (-a^2 - m^2)*b^2 - a^2*m^2)*n^2 + c^2*p^4 + (c^4 + (-a^2 - b^2 - m^2)*c^2 - a^2*m^2 + b^2*m^2)*p^2 + ((b^2 - m^2)*c^2 + m^2*(a^2 - b^2 + m^2))*a^2)*(a + b - c))/(a^4 - 2*a^2*b^2 - 2*a^2*c^2 + b^4 - 2*b^2*c^2 + c^4)})  coordinate (S)
			( {c/2},{c*(a^2 + b^2 - c^2)/(2*sqrt(-(a + b - c)*(a - b + c)*(a - b - c)*(a + b + c)))},{-1*(a^4*m^2 + 2*a^2*b^2*c^2 - a^2*b^2*m^2 - a^2*b^2*n^2 - a^2*c^2*m^2 - a^2*c^2*p^2 + b^4*n^2 - b^2*c^2*n^2 - b^2*c^2*p^2 + c^4*p^2)/(2*sqrt((a^4 - 2*a^2*b^2 - 2*a^2*c^2 + b^4 - 2*b^2*c^2 + c^4)*(a^4*m^2 + a^2*b^2*c^2 - a^2*b^2*m^2 - a^2*b^2*n^2 - a^2*c^2*m^2 - a^2*c^2*p^2 + a^2*m^4 - a^2*m^2*n^2 - a^2*m^2*p^2 + a^2*n^2*p^2 + b^4*n^2 - b^2*c^2*n^2 - b^2*c^2*p^2 - b^2*m^2*n^2 + b^2*m^2*p^2 + b^2*n^4 - b^2*n^2*p^2 + c^4*p^2 + c^2*m^2*n^2 - c^2*m^2*p^2 - c^2*n^2*p^2 + c^2*p^4)))})coordinate (T);
\pgfmathsetmacroFPU{\myr}{a*b*c/sqrt((b+c-a)* (a + b - c) *(a - b + c)* (a + b + c))}
\pgfmathsetmacroFPU{\myR}{sqrt((a^4*m^4 - 2*m^2*(b^2*n^2 + c^2*p^2)*a^2 + b^4*n^4 - 2*b^2*c^2*n^2*p^2 + c^4*p^4)/(a^4*m^2 + ((c^2 - m^2 - n^2)*b^2 + (-m^2 - p^2)*c^2 + m^4 + (-n^2 - p^2)*m^2 + n^2*p^2)*a^2 + b^4*n^2 + ((-n^2 - p^2)*c^2 + (-n^2 + p^2)*m^2 + n^4 - n^2*p^2)*b^2 + c^2*(p^2*c^2 + (n^2 - p^2)*m^2 - n^2*p^2 + p^4)))/2}
\pgfmathsetmacroFPU{\Angle}{acos(\myr/\myR)} 

\draw[tdplot_screen_coords] (T) circle [radius = \myR]; 
\draw [dashed] (A) -- (C) (S) --(G);
\draw (S) -- (A) (S) -- (B) (S) -- (C) (A) -- (B) -- (C);			
\draw (G) circle [radius = \myr];	
\end{scope}

 
		\path foreach \p/\g in {A/-90,B/-90,C/-90,S/90,G/-90,T/0}{(\p)node[c]{}+(\g:2.5mm) node{$\p$}};
	\end{tikzpicture}
\end{document}  
```

![image.png](/image?hash=69e8ef3e6e413c8cfffc9c1da3b0eaf021748b8aa6f56b7451acb1ea5bd059b2)

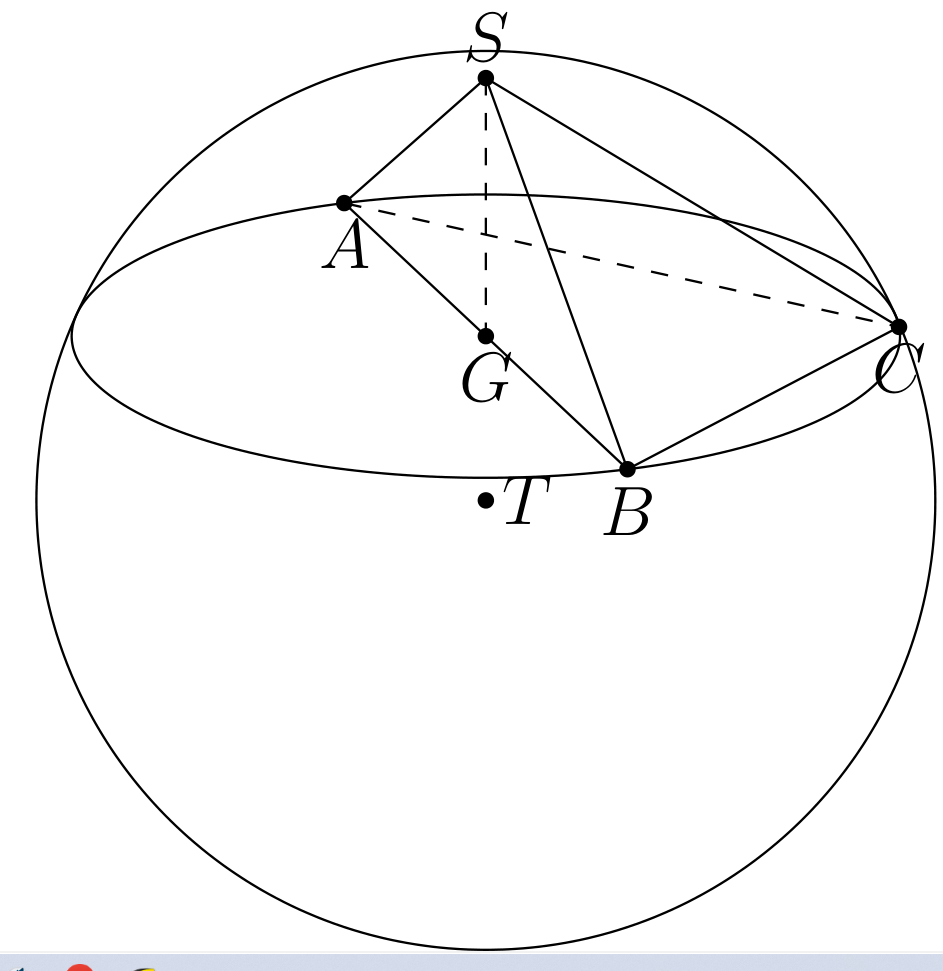
 I tried to draw a circle by using `tikz-3dplot-circleofsphere` (https://github.com/matthias-wolff/tikz-3dplot-circleofsphere). 
```
\begin{scope}[shift={(T)}]
 		\tdplotCsDrawLatCircle[blue, thick]{\myR}{\Angle}
 	\end{scope}
```

```
\documentclass[12pt]{standalone}
\usepackage{tikz,tikz-3dplot}
\usetikzlibrary{fpu,calc}
\usepackage{tikz-3dplot-circleofsphere}
\def\pgfmathsetmacroFPU#1#2{\begingroup%
	\pgfkeys{/pgf/fpu,/pgf/fpu/output format=fixed}%
	\pgfmathsetmacro{#1}{#2}%
	\pgfmathsmuggle#1\endgroup}%
\begin{document}
	\tdplotsetmaincoords{70}{70}
	\begin{tikzpicture}[tdplot_main_coords,line cap=butt,line join=round,c/.style={circle,fill,inner sep=1pt},
		declare function={a=3;b=4;c=5;m=3;n=3;p=3;}]
		\begin{scope}[/pgf/fpu,/pgf/fpu/output format=fixed]
			\path (0,0,0) coordinate (A)
			(c,0,0) coordinate (B)
			({(pow(b,2) + pow(c,2) - pow(a,2))/(2*c)},{sqrt((a+b-c) *(a-b+c) *(-a+b+c)* (a+b+c))/(2*c)},0) coordinate (C)
			(barycentric cs:A=1,B=1,C=1) coordinate (G)
			({(c^2 + m^2 - n^2)/(2*c)},{(-c^4 + (a^2 + b^2 + m^2 + n^2 - 2*p^2)*c^2 + (m - n)*(m + n)*(a - b)*(a + b))/(2*sqrt(-(a + b - c)*(a - b + c)*(a - b - c)*(a + b + c))*c)},{-1*sqrt((a - b + c)*(a - b - c)*(a + b + c)*(b^2*n^4 + ((a^2 - b^2 - c^2)*p^2 + (-b^2 + m^2)*c^2 + b^4 + (-a^2 - m^2)*b^2 - a^2*m^2)*n^2 + c^2*p^4 + (c^4 + (-a^2 - b^2 - m^2)*c^2 - a^2*m^2 + b^2*m^2)*p^2 + ((b^2 - m^2)*c^2 + m^2*(a^2 - b^2 + m^2))*a^2)*(a + b - c))/(a^4 - 2*a^2*b^2 - 2*a^2*c^2 + b^4 - 2*b^2*c^2 + c^4)})  coordinate (S)
			( {c/2},{c*(a^2 + b^2 - c^2)/(2*sqrt(-(a + b - c)*(a - b + c)*(a - b - c)*(a + b + c)))},{-1*(a^4*m^2 + 2*a^2*b^2*c^2 - a^2*b^2*m^2 - a^2*b^2*n^2 - a^2*c^2*m^2 - a^2*c^2*p^2 + b^4*n^2 - b^2*c^2*n^2 - b^2*c^2*p^2 + c^4*p^2)/(2*sqrt((a^4 - 2*a^2*b^2 - 2*a^2*c^2 + b^4 - 2*b^2*c^2 + c^4)*(a^4*m^2 + a^2*b^2*c^2 - a^2*b^2*m^2 - a^2*b^2*n^2 - a^2*c^2*m^2 - a^2*c^2*p^2 + a^2*m^4 - a^2*m^2*n^2 - a^2*m^2*p^2 + a^2*n^2*p^2 + b^4*n^2 - b^2*c^2*n^2 - b^2*c^2*p^2 - b^2*m^2*n^2 + b^2*m^2*p^2 + b^2*n^4 - b^2*n^2*p^2 + c^4*p^2 + c^2*m^2*n^2 - c^2*m^2*p^2 - c^2*n^2*p^2 + c^2*p^4)))})coordinate (T);
\pgfmathsetmacroFPU{\myr}{a*b*c/sqrt((b+c-a)* (a + b - c) *(a - b + c)* (a + b + c))}
\pgfmathsetmacroFPU{\myR}{sqrt((a^4*m^4 - 2*m^2*(b^2*n^2 + c^2*p^2)*a^2 + b^4*n^4 - 2*b^2*c^2*n^2*p^2 + c^4*p^4)/(a^4*m^2 + ((c^2 - m^2 - n^2)*b^2 + (-m^2 - p^2)*c^2 + m^4 + (-n^2 - p^2)*m^2 + n^2*p^2)*a^2 + b^4*n^2 + ((-n^2 - p^2)*c^2 + (-n^2 + p^2)*m^2 + n^4 - n^2*p^2)*b^2 + c^2*(p^2*c^2 + (n^2 - p^2)*m^2 - n^2*p^2 + p^4)))/2}
\pgfmathsetmacroFPU{\Angle}{acos(\myr/\myR)} 

\draw[tdplot_screen_coords] (T) circle [radius = \myR]; 
\draw [dashed] (A) -- (C) (S) --(G);
\draw (S) -- (A) (S) -- (B) (S) -- (C) (A) -- (B) -- (C);			

\end{scope}

 	\begin{scope}[shift={(T)}]
 		\tdplotCsDrawLatCircle[blue, thick]{\myR}{\Angle}
 	\end{scope}		
		\path foreach \p/\g in {A/-90,B/-90,C/-90,S/90,G/-90,T/0}{(\p)node[c]{}+(\g:2.5mm) node{$\p$}};
	\end{tikzpicture}
\end{document}  
```

![image.png](/image?hash=802be876ef291e50c7551ffdd7db0cfb012098bea00b91af7aa996edfb3544af)
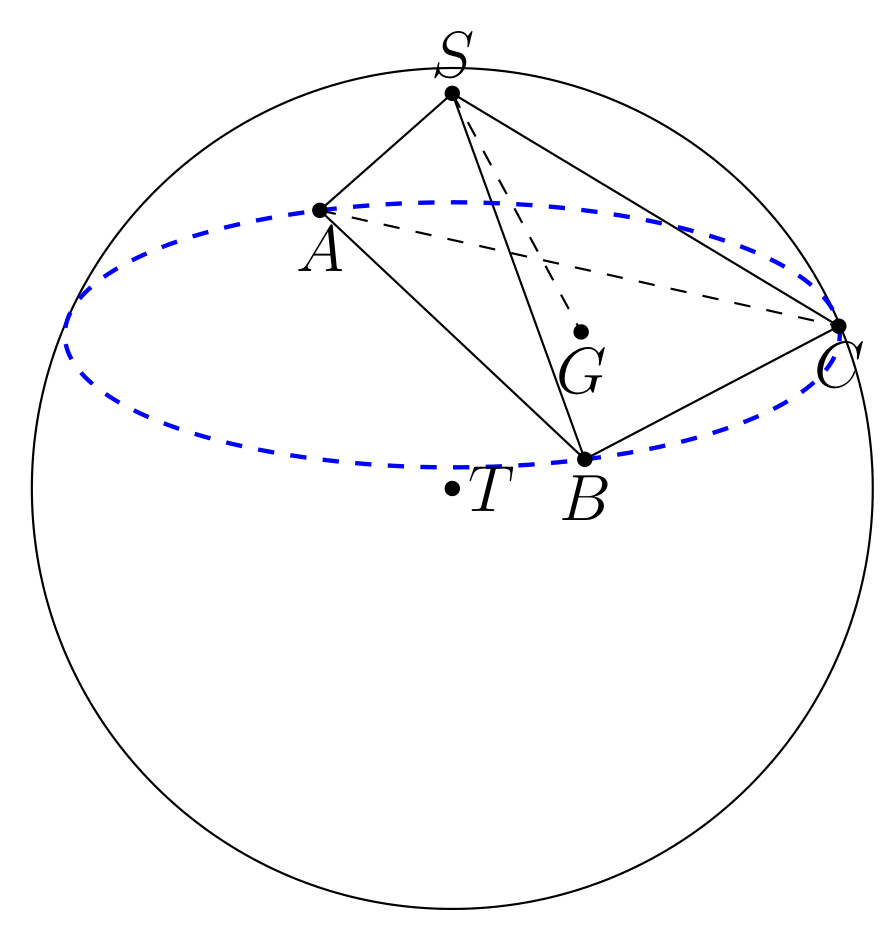
I got incorrect circle.